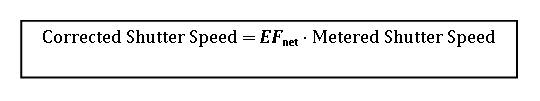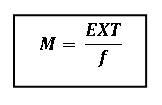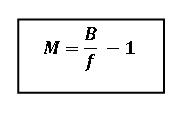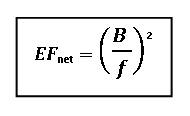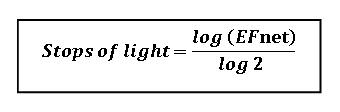In the final installment to this series, I have chosen to discuss one of my favorite topics in photography: close-ups. My goals with this article are to provide a basic understanding of light and exposure when photographing a subject at high magnification; to present a rationale for exposure density loss during magnification; and to provide guidance on how to correct for this exposure density loss.
To illustrate these principles, I will share my own empiric observations, review the pertinent calculations that govern magnification and exposure density loss compensation, and discuss select photographs that I have made at various magnifications.
Although I crafted this article from the framework of a photographer using traditional close-up and macro equipment (e.g., bellows, extension tubes), the use of an external light meter (i.e., non-TTL metering), and continuous lighting (e.g., natural light, lamps), the tenets and technical considerations presented here are still relevant to those photographers who prefer automation, TTL metering, and electronic flash.
Finally, I will wrap up the discussion by sharing some thoughts on the use of film as a tool for learning the visualization process.
The optics and exposure considerations in close-up photography are an interesting subject. Much has already been written on this topic over the decades. A discussion of the relevant principles may come across to the beginner as dry, cumbersome, and technically heavy-handed. Fortunately, a beginning or an advanced photographer need not understand all the technical details, since a fair amount of it has no bearing on real-world and practical close-up photography.
I have read three different textbooks on close-up and macro photography (published in the 1970s, 1980s, and mid-2000s). While each provides a superb and comprehensive treatment of this topic, I have found that the discussion of the rationale for the loss of exposure density at the image plane to be incomplete. Finding reliable literature on the internet on exposure compensation at high magnification can be disappointing, some of which is fraught with deficiencies, and quite frankly, incorrect interpretations. To date, one of the best books on macro photography that I have read that not only covers the problem of exposure loss at the film plane but also the approach to magnification, depth of field, and lighting is Lester Lefkowitz’s “The Manual of Close-Up Photography”, which I highly recommend.
“Don’t be creative when you should be mechanical, and don’t be mechanical when you should be creative.” – Fred Picker
The Problem
If there is one salient challenge in learning and experimenting with close-up and macro photography, it would be net exposure density loss at the film plane. This phenomenon determines everything from the choice of subjects to the choice of tools (e.g., the format, camera, lens, film, lighting, outdoor/indoor setting). Exposure density loss during the magnification of a subject at close range is inevitable and is based on time honored laws of optics and physics. So, the beginning photographer might ask: why is there net exposure density loss at the image plane in close-up and macro photography? Well, I am glad that you asked!
Let’s consider two simplified scenarios. First, the time honored overhead transparency projector. Have you ever noticed that by pulling the projector (if situated atop a rolling stand) away from the projecting screen causes the transparency to be magnified on the screen, dims the brightness on the screen, and causes the transparency to blur? And have you ever noticed that after moving the projector away that by turning that big plastic knob to lower the lens causes the projected, dimmed, and magnified image to pop into focus? Well, those observations epitomize the behavior of light and exposure in close-up photography.
Secondly, consider a basic SLR camera with an attached macro lens. No doubt, a photographer will have noticed that as he/she rotates the lens ring to set a higher magnification, the viewfinder progressively dims. And if that camera happens to be an advanced model with TTL metering, the astute photographer will also have observed that the LED light meter read-out (or analog meter needle in older models) in the viewfinder indicates a progressively longer exposure (i.e., a longer shutter speed) at the given aperture and ISO under the same scene luminance. Hmm … why a longer exposure?
From a conceptual and technical standpoint, the answer to why is straightforward and predicated on two competing physical properties: (1) light density *loss* due to the magnification effect and (2) light density *gain* due to the close focusing effect. Both effects are governed by the Inverse Square Law, which is the classic law of physics that holds that the intensity of light (energy per unit area) emanating from a point light source is inversely proportional to the square of the distance from the light source at that point in space and time.
For example, if you double the distance of a point from the light source, the light intensity per unit area at that point drops by one-fourth; conversely, if you halve the distance to the light source, the light intensity per unit area at that point quadruples. Pure and simple.

How is an object magnified in the first place? In order to magnify an object at the film plane, the lens plane must be moved away from the film plane (or vice versa). This is where it all starts. Intuitively, based on the physics of the Inverse Square Law, if light is allowed to travel farther from the lens (i.e., via extension) to form the image circle, then the image circle should enlarge, accompanied by a proportional enlargement of the projected subject. With greater distance between the lens and the film planes, the same quantity of light that enters the aperture of the lens is now spread out over a larger image circle (i.e., larger surface area).
Assuming that the scene luminance, aperture, and shutter speed (the three determinants of exposure) remain constant, since the same quantity of light now illuminates a larger image circle, the intensity of light (energy per unit area) at the image plane is diminished.
In essence, there is no absolute exposure loss during magnification, but there is a loss of exposure density (defined as light intensity per unit area per unit time) at the film plane.
Furthermore, once the subject is magnified, in order to maintain focus on the subject and thus obey the thin lens equation, the lens must be moved toward the subject. Intuitively, based on the physics of the Inverse Square Law, if the lens is moved closer to the light source (i.e., the subject), the intensity of light entering the aperture of the lens is now greater due to the decreased distance to the lens. Thus, the intensity of light (per unit area) that is destined to expose the film has increased.
In terms of creating the net exposure density on the film, which exposure effect reigns supreme – magnification or close-focusing? It turns out (both experimentally and mathematically) that the loss of exposure density at the film plane due to magnification exceeds the gain of exposure density due to close focusing, resulting in a net loss of exposure density at the film plane. For the photographer, this means that magnification automatically results in underexposure at the film plane. Pure and simple. If there is one take-away message from this article, this is it!
The ramification of this net loss of exposure density is enormous. If the photographer (using traditional manual equipment) does not take action to nullify this exposure density loss, then the result is disastrous: a guaranteed underexposed negative or transparency (gasp!) and a potentially ruined photograph. For those photographers who use automated cameras with TTL metering, this exposure density loss is never a concern, because the camera’s light meter will automatically adjust for the exposure density loss and render the correct shutter speed based on the set aperture and ISO. But for photographers using cameras with non-automated extension tubes or bellows (or both) where TTL metering is not possible, he/she must use an external light meter and apply an appropriate exposure adjustment (most commonly an adjustment in the shutter speed) in order to properly expose the film.
With this background set forth for the net exposure density loss due to magnification, the immediate practical questions become: (1) how much exposure density is lost and (2) how does the photographer compensate for it?
Consider the following demonstration that is not unlike the transparency projector analogy above. Using a large format view camera, I made a series of test exposures under controlled conditions (Figure 2). I manually extended the bellows of the camera to progressively increasing lengths; adjusted the focus on the subject; made test exposures under identical conditions (e.g., equivalent scene luminance, aperture, and shutter speed); and qualitatively compared the exposures.
I used an 8×10 field camera mounted with a Schneider G-Claron 150 mm f/9 lens. At infinity focus, this lens only covers the 4×5 format; it will heavily vignette when used with larger formats if it is focused at infinity. I chose this lens by design, for reasons that will be apparent. For my test subject, I used a paper template of a circle (16 cm diameter) affixed to a wall.
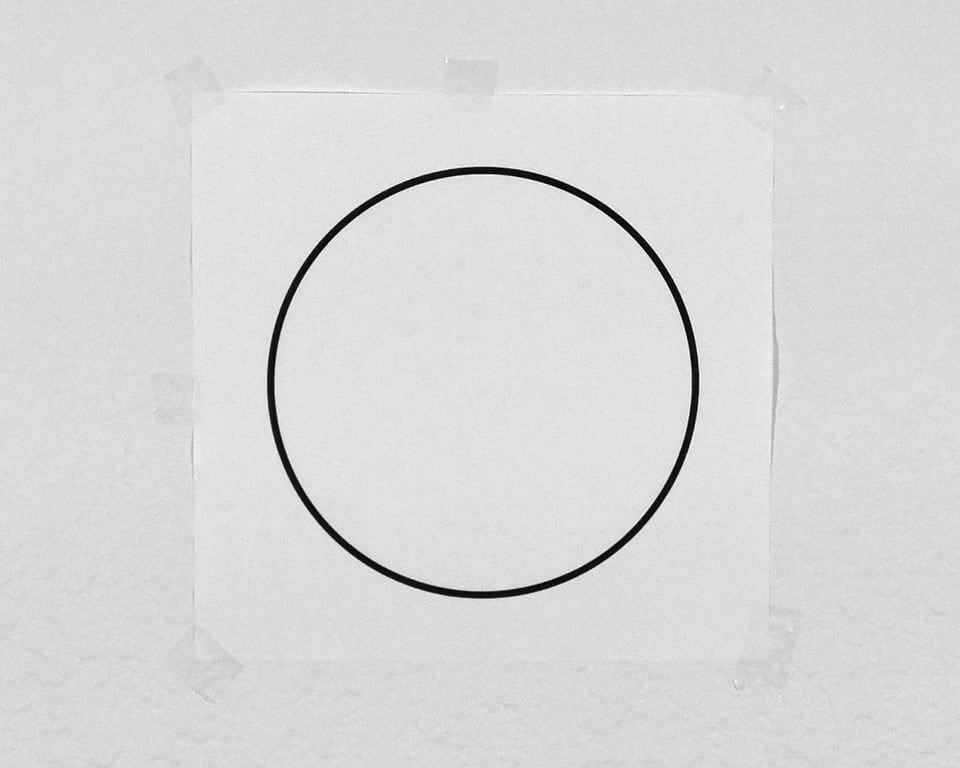
I made all exposures using the same light intensity on the wall (EV 11) provided by dual and symmetrical photoflood lamps; the same film (Kodak Ektascan BR/A); the same camera and lens at the same aperture (f/9); and the same shutter speed (¼ second). For all exposures, I spot metered the center of the brightly lit circle on Zone VIII. I developed all four negatives under the same conditions (“normal” development, 7 minute base).
- Figure 2A: Exposure made with the lens focused at infinity (i.e., bellows extended to 150 mm in length) with a subject distance of approximately 5 feet (10x the focal length).
- Figure 2B: Exposure made with the lens moved away from the film plane from infinity (bellows extended to 170 mm length) followed by moving the camera toward the subject to achieve focus.
- Figure 2C: Exposure made with the lens moved farther from the film plane from infinity (bellows extended to 225 mm in length) followed by moving the camera more toward the subject to achieve focus.
- Figure 2D: Exposure made with the lens moved even farther from the film plane from infinity (bellows extended to 300 mm in length) followed by moving the camera even closer to the subject to achieve focus.
Qualitatively, as I extended the bellows to achieve magnification, the brightness of the focusing screen (i.e., the ground glass) progressively dimmed, as expected. As you closely study all four negatives displayed together on the light box, what do you notice? Here is what has happened: as the lens is progressively moved away from the film plane by extending the bellows, (1) there is less vignetting; (2) the subject enlarges; and (3) the exposure density (per unit area) on the negative spreads out and markedly diminishes. This rough experiment demonstrates that as the lens is extended from the film plane to magnify the subject (followed by moving the camera toward the subject to achieve focus), the image circle enlarges, the subject is magnified, and there is net exposure density loss at the film plane. Again, since all exposures were identical (e.g., equivalent scene luminance, aperture, and shutter speed), there was no absolute exposure loss during magnification.
Furthermore, Figure 2 demonstrates that as the lens is extended, a point emerges where the image circle covers the entire rectangular image plane. An interesting (and practical) corollary is that a lens that does not have sufficient coverage for a given format at infinity may actually cover the format at high magnification (more on this later).
Next, I took the above experiment a step further in an attempt to crudely quantitate the exposure density loss. Just before I made the above exposures, I had taken spot light meter readings from the center of the ground glass focusing screen (the exact plane where the film will be exposed) for each lens extension with the focus adjusted (i.e., the lens-subject distance). The results, including the magnification for each lens extension, are summarized in Table 1:
| Figure | Bellows Extension (mm) | Subject Distance | Ground Glass Center Light Intensity (EV) | Magnification |
|---|---|---|---|---|
| Table 1: Light Intensity at Ground Glass With Lens Extended *and* Subject Focused | ||||
| 2A | 150 (infinity) | 5.0 feet | 6.1 | 0 |
| 2B | 170 | 4.2 feet | 5.2 | 13% (1:7.5) |
| 2C | 225 | 1.48 feet (450mm) | 5.1 | 50% (1:2) |
| 2D | 300 | 11.8 inches (300mm) | 4.1 | 100% (1:1) |
Based on this experiment, the Inverse Square Law’s net effect of lens extension from the film plane (magnification) and moving the lens toward the subject (close focusing) is manifest: substantial exposure density loss at the image plane.
But wait… we can draw more conclusions! In the special case of going from zero magnification (i.e., infinity focus) to 1:1 magnification, we see that (1) the requisite bellows extension (i.e., the extension past infinity focus plus the focal length) equals the subject distance; (2) the requisite bellows extension is equal to twice the focal length; and (3) the net exposure density loss appears to be equal to a whopping two stops. The plot thickens . . .
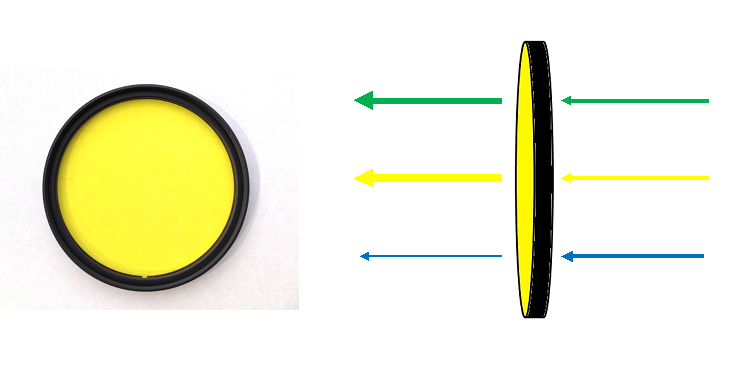
With these empiric observations, can the above conclusions be proven mathematically? I am thrilled that you asked that question! The quantity of exposure density lost at the film plane – and thus that which must be compensated during the exposure – and the interrelationships between magnification, lens extension, and focal length can all be elegantly derived from the Inverse Square Law, the thin lens model, and basic geometric and algebraic manipulations. The net Exposure Factor is simply the product of the two opposing factors – magnification and close focusing – that result in exposure density loss and exposure density gain, respectively, at the film plane. In the interest of brevity, you can view the derivation here. The end result is shown in Equation 1:
Eq 1
where M is the magnification. For those photographers who use bellows as their extension devices, in particular within the large format community, this exposure factor is also referred to as the “bellows factor”.
As this relationship shows, even a small increase in magnification results in an significant loss of exposure density (light intensity per unit area per unit time) at the film plane. In the special case of a life size magnification (1:1), the Exposure Factor becomes 4x, or two stops of light. Intuitively, this means that since the exposure density at 1:1 drops by ¼, the surface area of the image circle must be four times larger than it is at infinity, and the diameter of the image circle must be twice than it is at infinity.
The Exposure Factor equation is valid for any and all camera formats (e.g., film, digital, 35mm, medium format, large format) using any and all types of extension devices. From a practical standpoint, when the photographer wishes to achieve a 100% magnification, the shutter speed indicated by the external light meter must be multiplied by a factor of four to obtain the corrected shutter speed that will compensate for the exposure density loss at the image plane.
Alternatively, the photographer may wish to convert this Exposure Factor into stops of light (see below) and then apply this conversion to the external light meter by adjusting the ISO dial downward (I usually do this) to indicate additional exposure, not unlike how a photographer compensates for lens filter factors by adjusting the ISO dial downward.
There a few additional formulas that are instrumental to the close-up photographer using traditional macro equipment and an external light meter. The derivations may be viewed here. Expressed in terms of the Extension of the lens from the film plane (relative to infinity focus), the formula for magnification becomes:
Eq 2
where EXT is the length that the lens is extended from infinity focus and f is the focal length. Thus, these are the only two variables that dictate magnification: the amount of available lens extension and the focal length in use. Intuitively, what this expression tells us is that by allowing the light to travel farther between the lens and image planes, the projected image circle will enlarge. In practice, this extension may be achieved with the use of extension tubes, bellows, or a combination thereof.
In the case of a camera using solely a bellows attachment directly between the film plane and the lens, when the lens is focused at infinity by definition the bellows length equals the focal length. Subsequently, when the lens is extended from infinity to magnify, the bellows length is the sum of the focal length and the extension of the lens past infinity focus (B = EXT + f). With additional algebra, the magnification becomes:
In the special case of a life size magnification (1:1), Equations 2 and 3 give us the following practical corollaries, as suggested by the above experiments. The lens (which is already focused at infinity) must be extended from the film plane by a distance that is equal to the focal length. And for photographers using bellows alone, this is equivalent to extending the lens by a bellows length that is equal to twice the focal length.
Expressed in terms of the bellows length, with more algebraic manipulations the Exposure Factor correction becomes:
Intuitively, what this expression tells us is that by allowing the light to travel farther between the lens and the image plane to magnify, the exposure density loss to be compensated varies with the square of that distance (i.e., the extension). The Inverse Square Law!
Finally, for those photographers who prefer to use stops of light when compensating for exposure loss, the Exposure Factor can readily be converted as follows:
To summarize, a photographer needs only one (or two) variables to calculate the Exposure Factor: the desired magnification using Equation 1, or the lens extension available and the focal length using Equation 2 or Equation 3. Ordinarily, a close-up photographer does not need to calculate a precise magnification (especially in the field). I usually don’t, unless in the special case where I wish to make a 1:1 reproduction.
If there is one salient take home message from both the empiric observations above and these formulas, it is that lens extension is responsible for both magnification and the loss of exposure density and that the physical rationale for these phenomena is based on the Inverse Square Law.
In numerous other publications on exposure compensation in close-up photography, you will invariably come across a discussion of this problem conceptualized and mathematically expressed in terms of the “effective aperture”, “effective f-stop”, and “effective focal length” followed by a rather boring discussion of pupillary magnification. What these discussions refer to is that due to the loss of exposure at the film plane, the actual physical lens aperture behaves “as if” it has a smaller working aperture during magnification, “allowing less light” to expose the film. Of course, the physical size of the aperture does *NOT* change (although the calculated effective f-stop does), and the quantity of light passing through the aperture is constant (unless the photographer physically changes the intensity of the light source, the aperture, or the shutter speed). If the photographer prefers this approach, then he/she may calculate the “effective f-stop” (see derivation and sample calculation here) and use that value to set the external light meter to obtain the corrected shutter speed for the exposure.
Although the concept of “effective aperture” can be (somewhat) helpful for rationalizing the loss of exposure density at the film plane, potentially it may lead to confusion in understanding the true rationale (it’s the Inverse Square Law!) as well as a pointless fixation on aperture “equivalencies” and is best avoided, in my humble opinion. Remember: the reasons for the loss of exposure density are that light must travel farther to the image plane and the light intensity per unit area falls off precipitously with the square of the lens extension, not because the aperture physically gets smaller.
Furthermore, if the photographer intends to use the Exposure Factor to calculate a new working aperture to compensate for the loss of exposure density (that is, physically opening up the aperture in direct proportion to the Exposure Factor), then two problems readily occur: loss of depth of field with a wider aperture (which is already a problem as it is in close-ups) and complicating the problem of compensating for reciprocity departure for a long exposure. To simplify the conceptualization and approach to compensating for the exposure density loss, I would recommend to avoid the “effective f-stop” in the Exposure Factor calculation and stick to the lens extension/bellows or magnification – real physical properties that are aligned with the Inverse Square Law – to obtain the corrected shutter speed.
The mathematical formulas for exposure correction and magnification notwithstanding, an additional practical consideration is to know in advance the subject-lens distance threshold for which exposure compensation must be given. The practical “rule of thumb” is that for subject distances that are greater than nine times the focal length of the lens, no exposure compensation is needed. For subject distances that are at, or less, than this threshold distance, exposure compensation must be applied. You can view the mathematical derivation of this rule here. Essentially, this rule is based on the need to compensate for at least +⅓ stop additional exposure due to loss of exposure density from magnification and corresponds approximately to a minimum magnification of 12.5%, or a 1:8 reproduction ratio. The renowned and highly accomplished photographer, and one of the contemporary greats, Howard Bond, refers to this threshold distance as the “close-up triggering difference” in his article, “Exposure Corrections for Close-Ups”. Personally, I keep a check-list on hand of these threshold distances for all of my macro lenses for reference. When I am at, or within, this threshold distance, I automatically grab my tape ruler to measure the bellows length to calculate the exposure compensation.
There are other interesting (and practical) corollaries that are beyond the scope of this article but are worthwhile to mention briefly. Based on the thin lens equations for magnification and on real life photography, the choice of focal length can exert a powerful influence on how a photographer can achieve magnification based on his/her working restrictions and available tools on hand.
For example, if the photographer prefers to operate at a set “working distance” (closely approximated by the subject-lens distance) and does not wish to compromise this distance for technical or aesthetic reasons, then more magnification can be attained by simply using a lens of a longer focal length, provided of course, the photographer has the means to increase the lens extension.
Alternatively, if the photographer has a limited amount of lens extension with which to work but does not care about the working distance, then increasing magnification can be achieved by using a lens of a shorter focal length, but this of course would necessitate moving the lens even closer to the subject. For those interested, you can see a mathematical rationale here.
Furthermore, although depth of field in close-up and macro photography merits an article in its own right, many beginning close-up photographers may be surprised to learn that at the same magnification and at the same aperture, depth of field is independent of the focal length. That is, at the same magnification and aperture, a long focal length lens and a short focal length lens have the same depth of field. For real (and superb) examples of these interesting scenarios, I would recommend reading Lester Lefkowitz’s aforementioned text.
“Don’t be creative when you should be mechanical, and don’t be mechanical when you should be creative.”
Ok, enough of the empiric and theoretical discussion, let’s turn to a few examples of exposure compensation in close-up photography. In the scout shot below, I chose this fern to study and photograph at close range. What garnered my attention with this subject were the structure of its leaves, its soft shadows, and the potential for capturing fine detail at high magnification. This garden was enclosed within a shaded area. Under mostly clear skies in the afternoon, the scene was receiving a good amount of reflected light off concrete walls around the enclosure. The low-moderate contrast light lent itself well to capturing the lovely tonality and textures on the leaves.

In order to strengthen the contrast between the fern and the background soil, I sought to reduce the detail in the soil and darken it and simultaneously to lighten the tones of the fern leaves. To these ends, I used a relatively mild black and white contrast filter, the yellow Wratten #8. As discussed in Part II, a given color contrast filter strongly passes light whose wavelength lies adjacent to the color of the filter on the “color wheel”, thus allowing more exposure of that wavelength on the film. The yellow filter will pass a fair amount of yellow and green light to the film plane; if the exposure is sufficient, this passage can lighten the tones of the fern leaves. In general, out of all the wavelengths of light, many panchromatic black and white films are least sensitive to green light; if the objective is to lighten green foliage with a yellow filter, then the photographer would be wise to allow for ample exposure under the given lighting conditions. As discussed previously, since yellow filters block a fair amount blue light, which is responsible for illuminating shadows via skylight, they can be used to darken, or close, shadows. The use of a stronger filter, such an orange or a red filter, would have been too harsh; although either would have blocked substantially more blue light and closed the shadow detail in the background soil to a much greater degree, each would have darkened the green values – not lightened them – due of the position of red and orange on the color wheel.
For my camera and lens, I chose the Cambo SC 4×5 monorail view camera and my “go-to” lens, the Fujinon-A 240mm f/9, which is a compact, versatile, and sharp lens that can be used as a long-normal lens in the 4×5 format, a wide angle lens in the 8×10 format, and as a macro lens in either format. For film, I chose Ilford Delta 100 panchromatic black and white film.
As I do with all of my photographs, I used a composing card to study and select a composition. After I composed the subject to my liking, the framing suggested a magnification between 1:1 and 1:2 (a precise magnification was not necessary for this shot). To control depth of field, I closed the aperture to f/16⅓.
For metering, I again heavily relied on the Zone System. I spot metered one of the leaves on Zone V. Even though the long exposure would have automatically increased contrast by virtue of the high values receiving more exposure than the low values, I pre-visualized more contrast on the negative. Thus, in order to boost the high values on the leaves to expand the contrast range (i.e., negative expansion), I requested extended development of the film (N+1) from my lab technician.
Based on the subject-lens distance, I was well within nine focal lengths of my lens, meaning that I would need to compensate for exposure loss due to magnification. The bellows extension measured 41 cm, which gave me an Exposure Factor correction of 2.91x, which when converted to stops of light (and slightly rounded up to the nearest one-third stop) gave me approximately +1⅔ additional stops of light loss at the film plane for which to compensate. As a reminder, because negative film has a wide positive exposure latitude, slightly rounding up to the next one-third stop will have no adverse impact whatsoever on the exposure. Incidentally, the magnification was approximately 70%, or a 1 to 1.41 image scale, but I did not need to know this in advance.
With a chosen a film exposure index of 80 and the +⅓ stop filter factor for the Wratten #8, I dialed in these factors into the ISO dial of my spot light meter. The metered spot gave a shutter speed of 2 seconds at f/16⅓. Applying the Exposure Factor of +1⅔ stops to the metered shutter speed gives a corrected shutter speed of 6 seconds. Finally, accounting for the film reciprocity departure, the final shutter speed was 7 seconds (≈ +⅓ additional stop).
Upon studying the negative on the light box, the negative yielded good contrast, adequate sharpness, and good resolution. I personally scanned the negative on the Epson V850 and cropped the image to a 7:5 aspect ratio. The final image yielded exactly what I had visualized in my mind, visualized though my composing card, visualized on the ground glass, and finally on the negative: a pleasant tonal range with interesting structure and fine detail to explore.

1:1.41, Wratten #8 filter, N+1 development
For this next close-up example, I chose this palm tree to study and photograph. At first glance, I thought the subject might be too prosaic to explore, but as I studied it with my composing card, I found that the back-lighting to the right of the tree was creating alluring contrast and revealing interesting textures on the bark, all of which made this scene too good to pass up.

First, I used an SLR camera to make a photo at a conventional range (1:10 image scale) in order to capture more of the length of the subject in a natural perspective. At a 1:10 reproduction ratio, which corresponds to a subject-lens distance of 11x the focal length, the exposure density loss at the film plane is negligible and thus no exposure compensation is needed.

1:10, No filter, Normal development
For the next photograph, I used a view camera to make a magnified image of the same palm tree. Here, I used the Ikeda Anba 4×5, the Schneider G-Claron 150mm f/9, and a macro focusing rail, which can be an instrumental tool when adjusting fine focus at close-range. After setting up the tripod at the approximate subject distance, selecting a composition on the composing card and refining it on the ground glass, and using the focusing rail to adjust the focus, I selected a metering, exposure, and development strategy to give me that which I visualized on the final image: moderate contrast and tonality, fine detail and texture, and conveyed with an overall sense of abstraction.
I closed the aperture to f/22 to control depth of field. I metered the low values indicated by the “red circle” in the scout shot above and placed those on Zone III. The highest exposure value, indicated by the “white circle”, fell roughly in Zone VI. I desired to expand the contrast range of the scene from Zone III-Zone VI in the actual scene to Zone III-Zone VII on the negative, so I extended the development of the film by N+1.
The subject distance of 2 feet was well within nine focal lengths. The lens extension (bellows length) was 19 cm. The Exposure Factor was 1.6x, or approximately +⅔ stops. Instead of metering first and then applying the Exposure Factor to the shutter speed as I did in the previous example, I decided to dial in this factor into the ISO dial and then meter the scene. I used no filters. The metered spot gave a shutter speed of ⅓ seconds. At this shutter speed, film reciprocity departure is not applicable. I personally scanned the negative, made just a slight adjustment to the contrast in Photoshop, and cropped the image to a 5:4 aspect ratio.
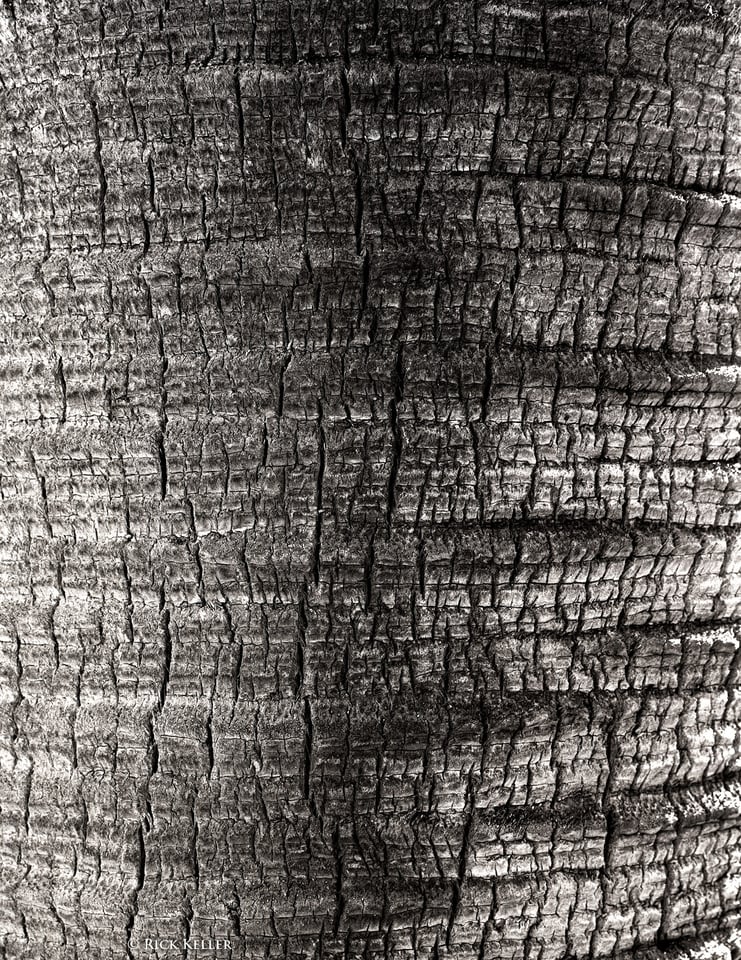
1:3.75, No filter, N+1 development
For this next close-up photograph, I chose a handful of rock salt to photograph at 1:1. Here, I chose a much smaller format, a 645 SLR camera, Kodak Tri-X panchromatic black and white film, and a dedicated macro lens. Like many modern SLR macro lenses, this particular lens has engraved magnification ratios on the barrel that allow the photographer to quickly and accurately set a specific magnification. Although this automated camera has TTL metering, I never use it. As I do with all of my cameras, whether manual or automated, I use an external hand held light meter for precise command and control of metering. For the lighting, I used a one photoflood lamp to the left and a silver reflector to the right for modest fill. With the lens barrel extended to 1:1, the focus adjusted with the macro rail, and the lighting set, I applied a two-stop exposure factor correction to my spot light meter, metered the scene, and then adjusted the shutter speed for reciprocity departure.

1:1, No filter, Normal development
For this next photo, I chose to make a table top close-up of a bouquet of flowers. I chose an 8×10 format with Ilford Delta 100 panchromatic black and white film. As I had demonstrated and briefly discussed earlier (see Figure 2A), the macro lens that I used for this photo, the G-Claron Schneider 150mm f/9, covers the 4×5 format at infinity (format diameter 165 mm, image circle 189 mm at infinity) but does not have enough coverage for the 8×10 format (format diameter 325 mm). However, as discussed above in the empiric and mathematical analyses, as the lens is extended to magnify, the image circle of the lens significantly enlarges. As I alluded to earlier, by definition, at 1:1 the image circle that the lens normally covers at infinity *doubles* in diameter. (See derivation here). Thus, at 1:1 the 150mm G-Claron has an image circle of 378 mm, enough to cover 8×10. For lighting, I used three photoflood lamps and two silver reflectors for fill (soft light from hard light!). Even though my magnification for this particular photograph was only 1:2, the lens still had enough covering power to make the photo, albeit with a tiny amount of vignetting at the corners, which was negligible and easily cropped off (see Figure 2C). Now, whether a given lens can perform well at both infinity and close range is another matter entirely. It just so happens that the G-Claron series represents a lens line-up that is optimized for 1:5 to 5:1, but they can also cover very well at infinity. And as this photo illustrates, the resolution and sharpness of this tiny lens at close range are quite good.

1:2, no filter, N-1 development
Finally, for this table top photograph of sunflowers, I again chose a smaller format, a 645 SLR camera, to make a modest 1:8 reproduction. At a subject distance of 9x the focal length, the exposure compensation for magnification was +⅓ stop. For the lighting, I used two photoflood tungsten lamps along with two silver reflectors for fill and strategically positioned them to deliberately create high contrast with deep shadows. Even at such a modest close range, what I enjoyed about this image was the strong contrast from this particular light set-up and the fine detail on the leaves and stems. Incidentally, the Mamiya 120mm f/4 Apo Macro manual focus lens is a *wickedly sharp* and contrasty macro lens that I highly recommend for medium format users.

1:8, No filter, N-1 development
Conclusions
The approach to compensating for the loss of exposure density at the image plane due to magnification of a close subject is straightforward and is based on time-honored and well understood principles of physics and optics. Magnifying a subject requires extending the lens from the film plane. An increase in magnification inexorably results in a predictable loss of exposure density at the image plane. Both the loss of exposure density (light intensity per unit area) and the increase in magnification are based on the Inverse Square Law, which is the fundamental principle that governs light intensity fall off with distance. Due to the loss of exposure density, in order to achieve a proper exposure density on the film the photographer using manual non-automated equipment must compensate for it by applying an an equivalent amount of additional exposure to that indicated by a light meter. The exposure correction is directly proportional to the square of the lens extension in use and directly proportional to the square of the magnification. That “square” is there for a reason . . .
Regarding the use of film as a tool in learning to visualize and make a good photograph, well, an entire article can surely be dedicated to this. Here are my thoughts. There are a plethora of aesthetic and technical reasons why a photographer would choose film as a tool and artistic medium. Many film photographers cite the emotion that film evokes, the look and feel of film, its color, quality, resolution, the wide variety of tools and formats from which to choose, the unique qualities of film stocks, and so forth, as to why they gravitate toward it. I actually share all of those sentiments, but those are not the reasons why I use film as a canvas for my own style of visualization. If there is one common denominator, one attribute, that epitomizes the beauty of film in visualization, it would be the *process*. As I hope this series of articles illustrated, the process of using film to learn and master visualization encompasses many considerations and behaviors that lend themselves well to constructing a photograph. Chief among these behaviors, shooting film makes the photographer slow down in just about every aspect of making a photograph, from visualizing the image in the mind, to exploring and waiting for the right light and emotion to materialize, to crafting the composition, to making the exposure, to developing the film, and to making a print. Every step along this aesthetic and technical journey involves, if not demands, some degree of forethought. In other words, film hones the photographer to systematically take command and control of every step. Period. I would argue that a more deliberate and contemplative approach to visualization should lead to the creation of stronger and more compelling photographs. Indeed, the contemplative film photographer makes fewer photographs, but those fewer attempts have more purpose in them. Being slow and deliberate is a positive thing for growth as a photographer and as an artist. Why the rush?
Further, in an uncanny manner the closing of the shutter and the wait to see the results immediately divorce the film photographer from the emotion, maybe the bias, of the moment so that when the time arrives for viewing the results on the light table, the photographer should have a clearer mindset to be a more objective critic of his/her work. That delay in seeing the end product is invaluable. The fun, the joy, and the triumphs of shooting film notwithstanding, the mistakes made in shooting film can be humbling, but those, too, are a good thing in the learning process. The permanence of the format, the ability to hold it in your hands and view both the triumphs and flaws in your decisions and technique, exert a powerful impact on this process.
Since I started film photography 4 years ago this month, I have shot dozens of rolls and sheets of film and have kept every single negative and transparency – both the good and the bad. I am not ashamed to admit that I have made a plethora of bad exposures on film – bad light, bad composition, and bad technique. Yet, I am resolute in turning my poor decisions to my advantage. I can make this happen because I never discard my film; and I never discard my bad photographs. The film, as a record, is always there with the photographer. There is no hiding from the truth . . .
Periodically, as an exercise in humility and in the spirit of honing my skill in visualization, I force myself to study my bad negatives all over again to review my mistakes and to refine my process of making a good photograph. Trust me, studying a bad photograph well after the fact – dissecting what was weak about it, questioning what I was feeling and seeing at the time – can be a humbling, unforgiving, and worthwhile experience all in one. More importantly, whether the exposure was good or bad, viewing it after being separated in space and time from the exposure can provide more motivation to ponder the meaning of the photograph and why the photographer made it. And that is what film ultimately does in this whole process: it keeps the photographer honest with himself.
Certainly, as a tool film is not well suited to every photographer’s style, comfort level, patience, or skill set; yet for the photographer who relishes the contemplative and deliberate thought process of constructing a photograph, I believe it is a tool that is worth exploring at least once in his/her life.
I hope you have enjoyed this series on film photography and visualization. Special thanks once again to Northcoast Photographic Services for providing the film development for these images. Great job, Scott! In future contributions to Photography Life in 2016, I envision sharing more experiences with light, composition, and various tools used in general photography (including travel photo essays from Southern California and specific film reviews) to help my colleagues out there, whether novice or advanced, young or old, to learn the art of this wonderful craft.
References
- “The Manual of Close-Up Photography”. Lester Lefkowitz.
- “Close-Ups in Nature”. John Shaw.
- “Close-Ups and Macro”. Robert Thompson.
- “Black and White Reciprocity Departure Revisited”. Howard Bond. Photo Techniques Magazine.
- “The Negative: The Ansel Adams Photography Series 2”. Ansel Adams.
- “The The Zone System Revisted Part I”. Popular Photography Magazine. Ansel Adams.
- “The Zone System Revisted Part II”. Popular Photography Magazine. Ansel Adams.
- “Gimme A Break”. Popular Photography Magazine. Russel Hart.
- “Zone VI Workshop”. Fred Picker.
- “Beyond The Zone System”. Phil Davis.
- “Scanning Tips”. Kenneth Lee Gallery
- “View Camera Magazine”
- “Principles of View Camera Focus”. Harold Merklinger.
- “View Camera Focus and Depth of Field: Part I”. Harold Merklinger.
- “Technical Books on Photography”. Harold Merklinger.
- “Check List for View Camera Users”. Howard Bond.
Table of Contents